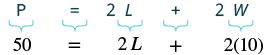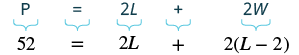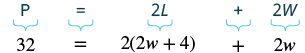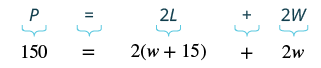Using the Properties of Rectangles to Solve Problems
Learning Outcomes
- Find perimeter and area of a rectangle given length and width
- Find dimensions of a rectangle given perimeter and a relationship between length and width
A rectangle has four sides and four right angles. The opposite sides of a rectangle are the same length. We refer to one side of the rectangle as the length, [latex]L[/latex], and the adjacent side as the width, [latex]W[/latex]. See the image below.
A rectangle has four sides, and four right angles. The sides are labeled L for length and W for width.
 The perimeter, [latex]P[/latex], of the rectangle is the distance around the rectangle. If you started at one corner and walked around the rectangle, you would walk [latex]L+W+L+W[/latex] units, or two lengths and two widths. The perimeter then is
[latex-display]\begin{array}{c}P=L+W+L+W\hfill \\ \hfill \text{or}\hfill \\ P=2L+2W\hfill \end{array}[/latex-display]
What about the area of a rectangle? Remember the rectangular rug from the beginning of this section. It was [latex]2[/latex] feet long by [latex]3[/latex] feet wide, and its area was [latex]6[/latex] square feet. See the image below. Since [latex]A=2\cdot 3[/latex], we see that the area, [latex]A[/latex], is the length, [latex]L[/latex], times the width, [latex]W[/latex], so the area of a rectangle is [latex]A=L\cdot W[/latex].
The area of this rectangular rug is [latex]6[/latex] square feet, its length times its width.
The perimeter, [latex]P[/latex], of the rectangle is the distance around the rectangle. If you started at one corner and walked around the rectangle, you would walk [latex]L+W+L+W[/latex] units, or two lengths and two widths. The perimeter then is
[latex-display]\begin{array}{c}P=L+W+L+W\hfill \\ \hfill \text{or}\hfill \\ P=2L+2W\hfill \end{array}[/latex-display]
What about the area of a rectangle? Remember the rectangular rug from the beginning of this section. It was [latex]2[/latex] feet long by [latex]3[/latex] feet wide, and its area was [latex]6[/latex] square feet. See the image below. Since [latex]A=2\cdot 3[/latex], we see that the area, [latex]A[/latex], is the length, [latex]L[/latex], times the width, [latex]W[/latex], so the area of a rectangle is [latex]A=L\cdot W[/latex].
The area of this rectangular rug is [latex]6[/latex] square feet, its length times its width.

Properties of Rectangles
- Rectangles have four sides and four right [latex]\left(\text{90}^ \circ\right)[/latex] angles.
- The lengths of opposite sides are equal.
- The perimeter, [latex]P[/latex], of a rectangle is the sum of twice the length and twice the width. See the first image. [latex]P=2L+2W[/latex]
- The area, [latex]A[/latex], of a rectangle is the length times the width. [latex]A=L\cdot W[/latex]
For easy reference as we work the examples in this section, we will restate the Problem Solving Strategy for Geometry Applications here.
Use a Problem Solving Strategy for Geometry Applications
- Read the problem and make sure you understand all the words and ideas. Draw the figure and label it with the given information.
- Identify what you are looking for.
- Name what you are looking for. Choose a variable to represent that quantity.
- Translate into an equation by writing the appropriate formula or model for the situation. Substitute in the given information.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete sentence.
try it
[ohm_question]146510[/ohm_question]
example
Find the length of a rectangle with perimeter [latex]50[/latex] inches and width [latex]10[/latex] inches.
Answer:
Solution
| Step 1. Read the problem. Draw the figure and label it with the given information. |
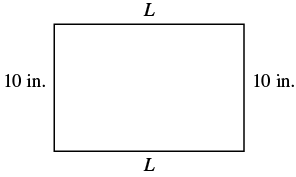 |
| Step 2. Identify what you are looking for. |
the length of the rectangle |
| Step 3. Name. Choose a variable to represent it. |
Let L = the length |
| Step 4. Translate.
Write the appropriate formula.
Substitute. |
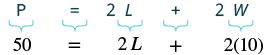 |
| Step 5. Solve the equation. |
[latex]50\color{red}{- 20}=2L+20\color{red}{- 20}[/latex]
[latex-display]30=2L[/latex-display]
[latex-display]\frac{30}{\color{red}{2}}=\frac{2L}{\color{red}{2}}[/latex-display]
[latex]15=L[/latex] |
| Step 6. Check:
[latex-display]p=50[/latex-display]
[latex-display]15+10+15+10\stackrel{?}{=}50[/latex-display]
[latex]50=50\checkmark[/latex] |
|
| Step 7. Answer the question. |
The length is [latex]15[/latex] inches. |
try it
[ohm_question]146518[/ohm_question]
In the next example, the width is defined in terms of the length. We’ll wait to draw the figure until we write an expression for the width so that we can label one side with that expression.
example
The width of a rectangle is two inches less than the length. The perimeter is [latex]52[/latex] inches. Find the length and width.
Answer:
Solution
| Step 1. Read the problem. |
|
| Step 2. Identify what you are looking for. |
the length and width of the rectangle |
| Step 3. Name. Choose a variable to represent it.
Now we can draw a figure using these expressions for the length and width. |
Since the width is defined in terms of the length, we let L = length. The width is two feet less that the length, so we let L − 2 = width
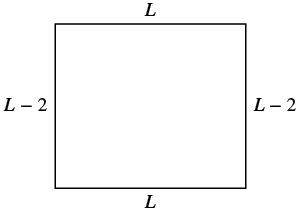 |
| Step 4.Translate.
Write the appropriate formula. The formula for the perimeter of a rectangle relates all the information.
Substitute in the given information. |
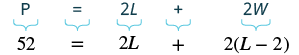 |
| Step 5. Solve the equation. |
[latex]52=2L+2L - 4[/latex] |
| Combine like terms. |
[latex]52=4L - 4[/latex] |
| Add [latex]4[/latex] to each side. |
[latex]56=4L[/latex] |
| Divide by [latex]4[/latex]. |
[latex]\frac{56}{4}=\frac{4L}{4}[/latex] |
|
[latex]14=L[/latex] |
|
The length is [latex]14[/latex] inches. |
| Now we need to find the width. |
[latex]L-2[/latex]
[latex-display]\color{red}{14}-2[/latex-display]
[latex]12[/latex] |
| The width is [latex]L−2[/latex]. |
The width is [latex]12[/latex] inches. |
| Step 6. Check:
Since [latex]14+12+14+12=52[/latex] , this works! |
|
| Step 7. Answer the question. |
The length is [latex]14[/latex] feet and the width is [latex]12[/latex] feet. |
try it
[ohm_question]146504[/ohm_question]
example
The length of a rectangle is four centimeters more than twice the width. The perimeter is [latex]32[/latex] centimeters. Find the length and width.
Answer:
Solution
| Step 1. Read the problem. |
|
| Step 2. Identify what you are looking for. |
the length and width |
| Step 3. Name. Choose a variable to represent it. |
let W = width
The length is four more than twice the width.
[latex]2w+4[/latex] = length
 |
| Step 4.Translate.
Write the appropriate formula and substitute in the given information. |
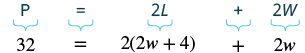 |
| Step 5. Solve the equation. |
[latex]32=4w+8+2w[/latex]
[latex-display]32=6w+8[/latex-display]
[latex-display]24=6w[/latex-display]
[latex]4+w[/latex] width
[latex]2w+4[/latex] length
[latex-display]2(\color{red}{4})+4[/latex-display]
[latex]12[/latex] The length is [latex]12[/latex]cm. |
| Step 6. Check:
[latex-display]p=2L+2W[/latex-display]
[latex-display]\stackrel{?}{=}2\cdot 12+2\cdot 4[/latex-display]
[latex]32=32[/latex] |
|
| Step 7. Answer the question. |
The length is [latex]12[/latex] cm and the width is [latex]4[/latex] cm. |
try it
[ohm_question]146521[/ohm_question]
[ohm_question]146522[/ohm_question]
example
The area of a rectangular room is [latex]168[/latex] square feet. The length is [latex]14[/latex] feet. What is the width?
Answer:
Solution
| Step 1. Read the problem. |
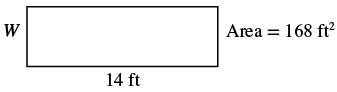 |
| Step 2. Identify what you are looking for. |
the width of a rectangular room |
| Step 3. Name. Choose a variable to represent it. |
Let W = width |
| Step 4.Translate.
Write the appropriate formula and substitute in the given information. |
[latex]A=LW[/latex]
[latex]168=14W[/latex] |
| Step 5. Solve the equation. |
[latex]\frac{168}{14}=\frac{14W}{14}[/latex]
[latex]12=W[/latex] |
| Step 6. Check:
[latex-display]A=LW[/latex-display]
[latex-display]168\stackrel{?}{=}14\cdot 12[/latex-display]
[latex]168=168\checkmark[/latex] |
|
| Step 7. Answer the question. |
The width of the room is [latex]12[/latex] feet. |
try it
[ohm_question]146523[/ohm_question]
example
The perimeter of a rectangular swimming pool is [latex]150[/latex] feet. The length is [latex]15[/latex] feet more than the width. Find the length and width.
Answer:
Solution
| Step 1. Read the problem. Draw the figure and label it with the given information. |
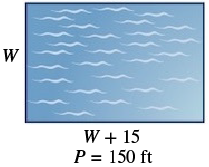 |
| Step 2. Identify what you are looking for. |
the length and width of the pool |
| Step 3. Name. Choose a variable to represent it.
The length is [latex]15[/latex] feet more than the width. |
Let [latex]W=\text{width}[/latex]
[latex]W+15=\text{length}[/latex] |
| Step 4.Translate.
Write the appropriate formula and substitute. |
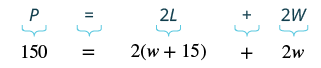 |
| Step 5. Solve the equation. |
[latex]150=2w+30+2w[/latex]
[latex-display]150=4w+30[/latex-display]
[latex-display]120=4w[/latex-display]
[latex]30=w[/latex] the width of the pool.
[latex]w+15[/latex] the length of the pool.
[latex-display]\color{red}{30}+15[/latex-display]
[latex]45[/latex] |
| Step 6. Check:
[latex-display]p=2L+2W[/latex-display]
[latex-display]150\stackrel{?}{=}2(45)+2(30)[/latex-display]
[latex]150=150\checkmark[/latex] |
|
| Step 7. Answer the question. |
The length of the pool is [latex]45[/latex] feet and the width is [latex]30[/latex] feet. |
try it
[ohm_question]146524[/ohm_question]
Licenses & Attributions
CC licensed content, Original
- Question ID 146524, 146522, 146518, 146504. Authored by: Lumen Learning. License: CC BY: Attribution.
CC licensed content, Specific attribution
 The perimeter, [latex]P[/latex], of the rectangle is the distance around the rectangle. If you started at one corner and walked around the rectangle, you would walk [latex]L+W+L+W[/latex] units, or two lengths and two widths. The perimeter then is
[latex-display]\begin{array}{c}P=L+W+L+W\hfill \\ \hfill \text{or}\hfill \\ P=2L+2W\hfill \end{array}[/latex-display]
What about the area of a rectangle? Remember the rectangular rug from the beginning of this section. It was [latex]2[/latex] feet long by [latex]3[/latex] feet wide, and its area was [latex]6[/latex] square feet. See the image below. Since [latex]A=2\cdot 3[/latex], we see that the area, [latex]A[/latex], is the length, [latex]L[/latex], times the width, [latex]W[/latex], so the area of a rectangle is [latex]A=L\cdot W[/latex].
The area of this rectangular rug is [latex]6[/latex] square feet, its length times its width.
The perimeter, [latex]P[/latex], of the rectangle is the distance around the rectangle. If you started at one corner and walked around the rectangle, you would walk [latex]L+W+L+W[/latex] units, or two lengths and two widths. The perimeter then is
[latex-display]\begin{array}{c}P=L+W+L+W\hfill \\ \hfill \text{or}\hfill \\ P=2L+2W\hfill \end{array}[/latex-display]
What about the area of a rectangle? Remember the rectangular rug from the beginning of this section. It was [latex]2[/latex] feet long by [latex]3[/latex] feet wide, and its area was [latex]6[/latex] square feet. See the image below. Since [latex]A=2\cdot 3[/latex], we see that the area, [latex]A[/latex], is the length, [latex]L[/latex], times the width, [latex]W[/latex], so the area of a rectangle is [latex]A=L\cdot W[/latex].
The area of this rectangular rug is [latex]6[/latex] square feet, its length times its width.