example
Determine whether each of the numbers in the following list is a 1. whole number, 2. integer, 3. rational number, 4. irrational number, and 5. real number.
[latex-display]-7,\Large\frac{14}{5}\normalsize ,8,\sqrt{5},5.9,-\sqrt{64}[/latex-display]
Solution:
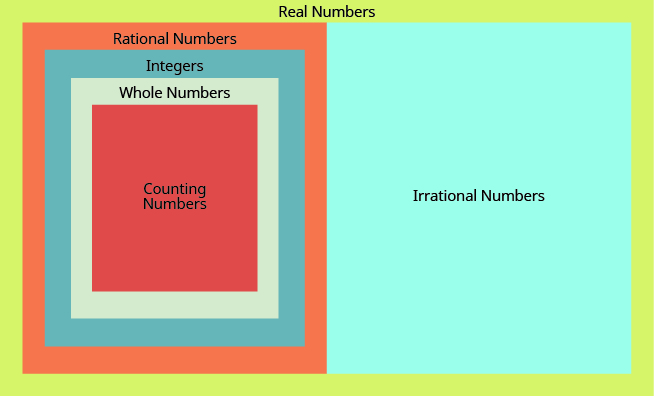
1. The whole numbers are [latex]0,1,2,3\dots[/latex] The number [latex]8[/latex] is the only whole number given.
2. The integers are the whole numbers, their opposites, and [latex]0[/latex]. From the given numbers, [latex]-7[/latex] and [latex]8[/latex] are integers. Also, notice that [latex]64[/latex] is the square of [latex]8[/latex] so [latex]-\sqrt{64}=-8[/latex]. So the integers are [latex]-7,8,-\sqrt{64}[/latex].
3. Since all integers are rational, the numbers [latex]-7,8,\text{and}-\sqrt{64}[/latex] are also rational. Rational numbers also include fractions and decimals that terminate or repeat, so [latex]\Large\frac{14}{5}\normalsize\text{and}5.9[/latex] are rational.
4. The number [latex]5[/latex] is not a perfect square, so [latex]\sqrt{5}[/latex] is irrational.
5. All of the numbers listed are real.
We'll summarize the results in a table.
| Number |
Whole |
Integer |
Rational |
Irrational |
Real |
| [latex]-7[/latex] |
|
[latex]\quad\checkmark [/latex] |
[latex]\quad\checkmark [/latex] |
|
[latex]\quad\checkmark [/latex] |
| [latex]\Large\frac{14}{5}[/latex] |
|
|
[latex]\quad\checkmark [/latex] |
|
[latex]\quad\checkmark [/latex] |
| [latex]8[/latex] |
[latex]\quad\checkmark [/latex] |
[latex]\quad\checkmark [/latex] |
[latex]\quad\checkmark [/latex] |
|
[latex]\quad\checkmark [/latex] |
| [latex]\sqrt{5}[/latex] |
|
|
|
[latex]\quad\checkmark [/latex] |
[latex]\quad\checkmark [/latex] |
| [latex]5.9[/latex] |
|
|
[latex]\quad\checkmark [/latex] |
|
[latex]\quad\checkmark [/latex] |
| [latex]-\sqrt{64}[/latex] |
|
[latex]\quad\checkmark [/latex] |
[latex]\quad\checkmark [/latex] |
|
[latex]\quad\checkmark [/latex] |